Essential insights:
- Uncover the power of Taylor VaR methodology and the benefits of its implementation. Efficient risk estimations provide a comprehensive breakdown of VaR drivers, enabling informed decision making beyond conventional revaluation approaches.
- Understand the challenges of Taylor VaR implementation by exploring analytical fundamentals and how to deploy them in conjunction with existing analytical frameworks to achieve deeper market vision.
- Discover Atoti, the technological solution for these challenges. ActiveViam’s cutting-edge Big Data Analytics platform revolutionizes Taylor VaR computations.
In today’s complex and diverse financial landscape, risk management is the foundational challenge of any organization’s operations. Financial institutions utilize many tools and methodologies to monitor and control the different risks they are exposed to. One of the most widely used measurement and management tools for quantifying and managing market risk associated with financial products is Value at Risk (VaR).
VaR provides a single figure that estimates the maximum potential loss that may be incurred during a specific period, under specific market conditions, with a given level of confidence. By using VaR, financial institutions can identify liabilities, establish risk limits, and make informed decisions about their portfolio’s composition.
While VaR offers great depth of insight into risk management profiles, it can also present an incomplete and insufficient picture of market fluctuations critical for informed decision making. Leadership needs metrics that deliver quick, clear predictive authority and confidence in the face of rapidly-evolving marketplace dynamics.
Taylor VaR is a lesser-known method of calculating VaR that can be a powerful tool for reimagining risk that achieves greater agility with minimal loss of precision. We will explore the benefits of adopting this approach while addressing the challenges that financial institutions may encounter during its implementation. Additionally, we will introduce Atoti, ActiveViam’s technology solution, which empowers firms to overcome these implementation hurdles and fully leverage the rewards of utilizing Taylor VaR.
What is Taylor VaR?
Taylor VaR has gained popularity within financial institutions due to its ability to estimate and deconstruct the nature of risks associated with its financial products. The approach is named after the Taylor series, an algorithm that finds several applications within the risk management realm such as profit and loss attribution calculations (PnL).
Taylor VaR algorithms rely on the use of sensitivities produced by the firm to estimate the value of its portfolios in response to various market movements, where each value estimation represents a specific scenario. These sensitivities include:
- delta (δ): the portfolio’s value change for a unit change in the underlying asset’s price
- gamma (γ): the change in delta for a unit change in the underlying asset’s price
- vega (v): the portfolio’s value change for a unit change in the underlying asset’s volatility
To simplify, let’s consider only delta and gamma for a given portfolio. The result of an approximate scenario is given by the following formula, where ΔS is the change in the portfolio’s underlying asset prices:
Once the desired number of marketplace scenarios has been modeled, the approximated returns are utilized as inputs for VaR calculations.
The interrogative value of Taylor VaR metrics
One of the advantages of Taylor VaR is its ability to expose the drivers of a full revaluation. It not only provides a clear understanding of the different risk factors that contribute to the portfolio’s VaR, but also allows users to focus on the tail of the distribution and see the PnL drivers behind the worst-case scenarios.
This more granular perspective enables risk managers to identify exposures that dominate in a worst-case scenario, something that is not possible with a full revaluation method (such as historical or parametric VaR) which only produces an overall figure.
By using Taylor VaR methodology, portfolio valuation can be both more transparently and efficiently derived by recomputing only those market factors in flux the existing priced PnLs.
Appropriate application of Taylor VaR analytics
The primary limitation of Taylor VaR algorithms is that it requires sensitivities to be based on assumptions imposed upon it by users. As a result, its predictive and modeling value offers precision that varies proportionally to the accuracy of those variables to real-world events, which places a greater analytical what-if burden on the quants selecting them.
As a result, outputs may be less accurate for complex products with non-linear payoffs, but it still produces VaR estimates that are nearly identical to those generated by full revaluation methods for simpler linear products. This makes it a faster and more efficient method for estimating VaR, particularly for large portfolios of linear products as their re-pricing is not required.
Challenges of implementing Taylor VaR analytics
Despite the benefits brought by Taylor VaR integration, financial institutions may encounter various actuation challenges. The computational intensity required and time-consuming nature of the calculations require highly-efficient and powerful software architecture, particularly when dealing with large portfolios. The calculation process for Taylor VaR involves handling a substantial number of sensitivities for the computation of the Taylor expansion.
Additionally, ensuring dynamic aggregation becomes crucial to enable flexible analysis across multiple dimensions, ranging from consolidated levels to detailed individual trades. Because the Taylor VaR analyses deliver speed and insight through granularity, it’s important to avoid shortcuts that rely solely on pre-aggregations, as this can limit flexibility in drilling down and filtering data without the need for lengthy batch computations.
To overcome these challenges, firms need to invest in the right tools and technologies that enable efficient Taylor VaR calculations, ensuring that risk managers make informed decisions in a timely manner. Without such tools, the process of aggregating and analyzing data across multiple dimensions (from the top of the firm, to the granular trade-level, and the even more granular risk factor-level) can become a bottleneck, slowing down the VaR estimation process and potentially leading to inaccurate results.
Revolutionizing Taylor VaR computation with Atoti
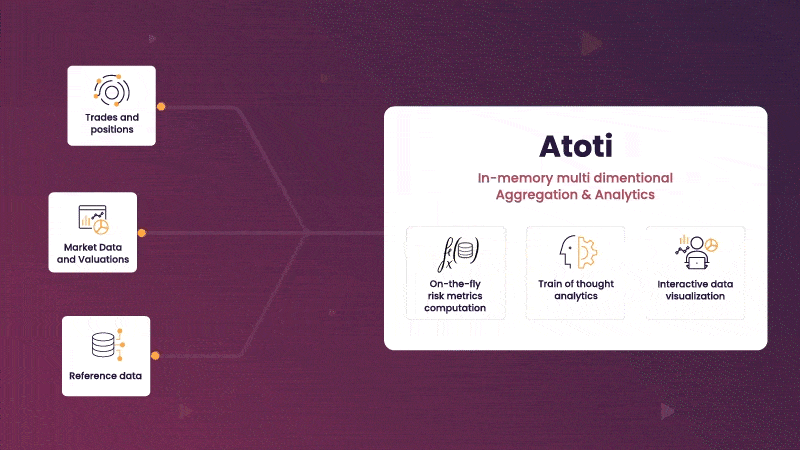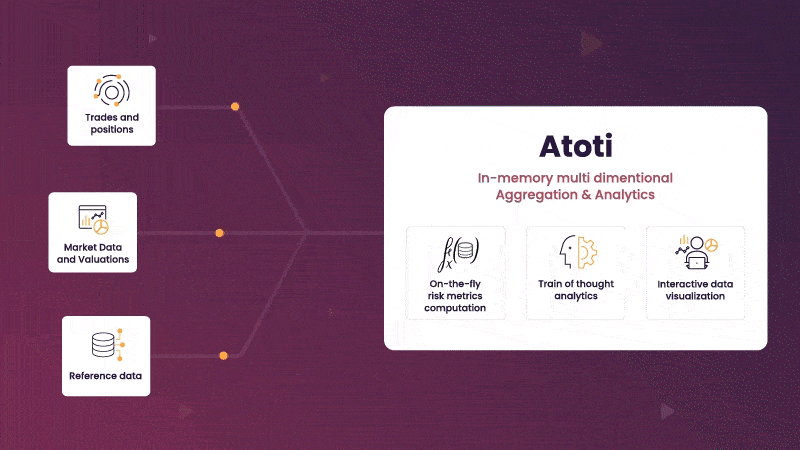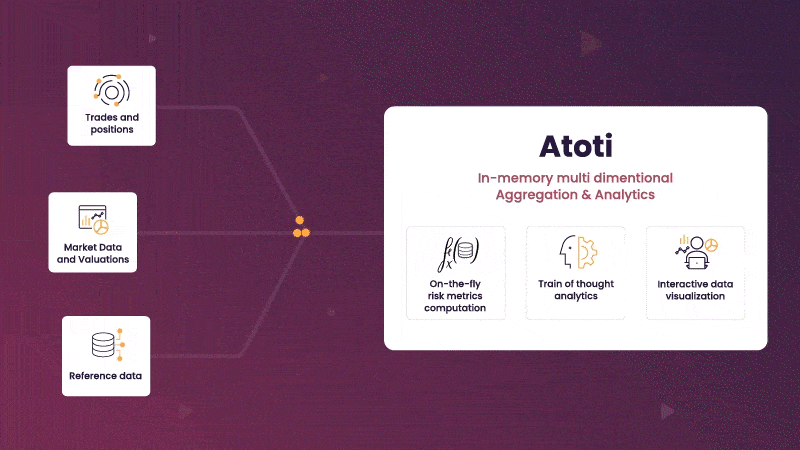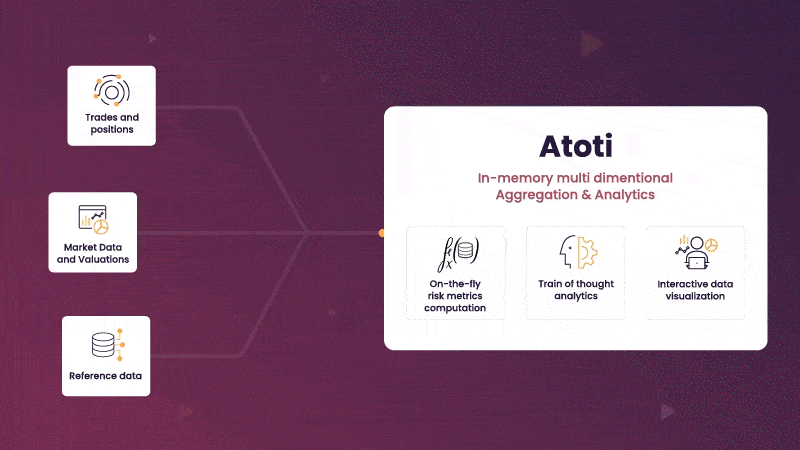
Atoti, ActiveViam’s cutting-edge risk management platform, provides financial institutions with a comprehensive solution to tackle the computational challenges of risk metrics through an efficient and flexible Big Data Analytics platform.
Atoti ingests streams of data produced in-house, including trade and position records and valuations, reference data depicting the firm’s structure (desks, books, and portfolio composition), and market data (raw data, sensitivities, etc.) required to perform risk calculations at the most granular level.
Through innovative analytical architecture and advanced data aggregation capabilities, Atoti is capable of processing and normalizing large and diverse data archives to facilitate complex risk calculations—including Taylor VaR—effortlessly and at scale. Its high-performance in-memory what-if capabilities empower users to leverage that data with extreme assumptive flexibility, from the top-level of the firm down to the granular trade level. This enables efficient analysis and decision making, providing users with valuable insights and a comprehensive view of their risk landscape.
Key benefits of Atoti’s Taylor VaR capabilities
1. Breaking Down Taylor VaR Multidimensional Aggregation
Watch the video with captions on our YouTube channel
With Atoti, analyzing Taylor VaR figures becomes effortless, providing a comprehensive breakdown of all analytical strata and eliminating the need for lengthy batch computations.
Atoti’s powerful in-memory aggregation capabilities enable seamless data exploration, eliminating delays and bottlenecks. Atoti instantly retrieves firm-level aggregated Taylor VaR figures, and dimensions are added on-the-fly to analyze results at desk-level, risk class-level, trade-level, and even down to the precise risk factor-level.
2. Leveraging Approximation Metrics to Improve PnL Observations
Watch the video with captions on our YouTube channel
In the fast-paced world of market risk management, timely understanding of the drivers behind tail scenarios is crucial. Atoti’s Taylor VaR capabilities provide users with unparalleled transparency into deep layers of analytical complexity, to reveal observational errors in PnL observations that would previously go unnoticed.
Using Atoti’s powerful data visualization capabilities, our risk manager analyzes the PnL distribution that serves as the basis for computing Taylor VaR. This distribution comprises 250 scenarios, each with PnL observations and their corresponding Taylor approximations. With a few clicks, risk managers can quickly identify the worst-case scenarios and focus resources on the most negative PnL observation.
As risk managers interrogate the problem, Atoti seamlessly guides them through the data, providing a granular view of the risk classes contributing to this scenario. Taylor analysis approximation measures can be applied on the fly, revealing proximate delta, gamma, and vega stressors in the worst-case scenario.
This allows risk managers to explain poor performance to leadership rather than simply identify it, which results in better decision making, streamlined strategic thinking, and greater corrective synergy between quants and leadership in all aspects of risk management.
3. VaR Explain Functionality With Atoti
Watch the video with captions on our YouTube channel
Atoti provides risk managers with all the tools required to perform “VaR Explain”, an analysis that enables them to easily test the accuracy of the firm’s Taylor VaR model.
Because Taylor VaR analysis provides a much faster and variable-specific perspective on VaR, it necessarily incorporates a degree of imprecision. It’s critical for risk managers to understand the tolerances of their modeling, and Atoti’s VaR Explain function they can pinpoint areas where the Taylor VaR model may deviate from the full revaluation approach, providing valuable insights into the sources of risk not captured by the model.
Analysis of Atoti’s Taylor VaR modeling capabilities reveals that 97% of full revaluation VaR can be explained, highlighting its robustness. Risk managers can easily identify desks with high unexplained portions of VaR and drill down into the data for further investigation.
The VaR Explain analysis not only serves as a catalyst for model improvements and refinements in risk management strategies, but it also empowers risk managers with the necessary insights to evaluate and enhance VaR computations throughout the firm. With Atoti’s powerful visualization capabilities at their disposal, risk managers can confidently assess their models, make well-informed decisions, and drive continuous improvements in their risk management practice.